Practical Kullback-Leibler (KL) Divergence: Discrete Case

KL divergence (Kullback-Leibler57) or KL distance is non-symmetric measure of difference between two probability distributions. It is related to mutual information and can be used to measure the association between two random variables.

In this short tutorial, I show how to compute KL divergence and mutual information for two categorical variables, interpreted as discrete random variables.

${\bf Definition}$: Kullback-Leibler (KL) Distance on Discrete Distributions

Figure: Distance between two distributions. (Wikipedia)

Given two discrete probability distributions ${\it P}(A)$ and ${\it Q}(B)$ with discrete random variates, $A$ and $B$, having realisations $A=a_{j}$ and $B=b_{j}$, over $n$ singletons $j=1,...,n$. KL divergence or distance $D_{KL}$ in between $P$ and $Q$ is defined as follows:

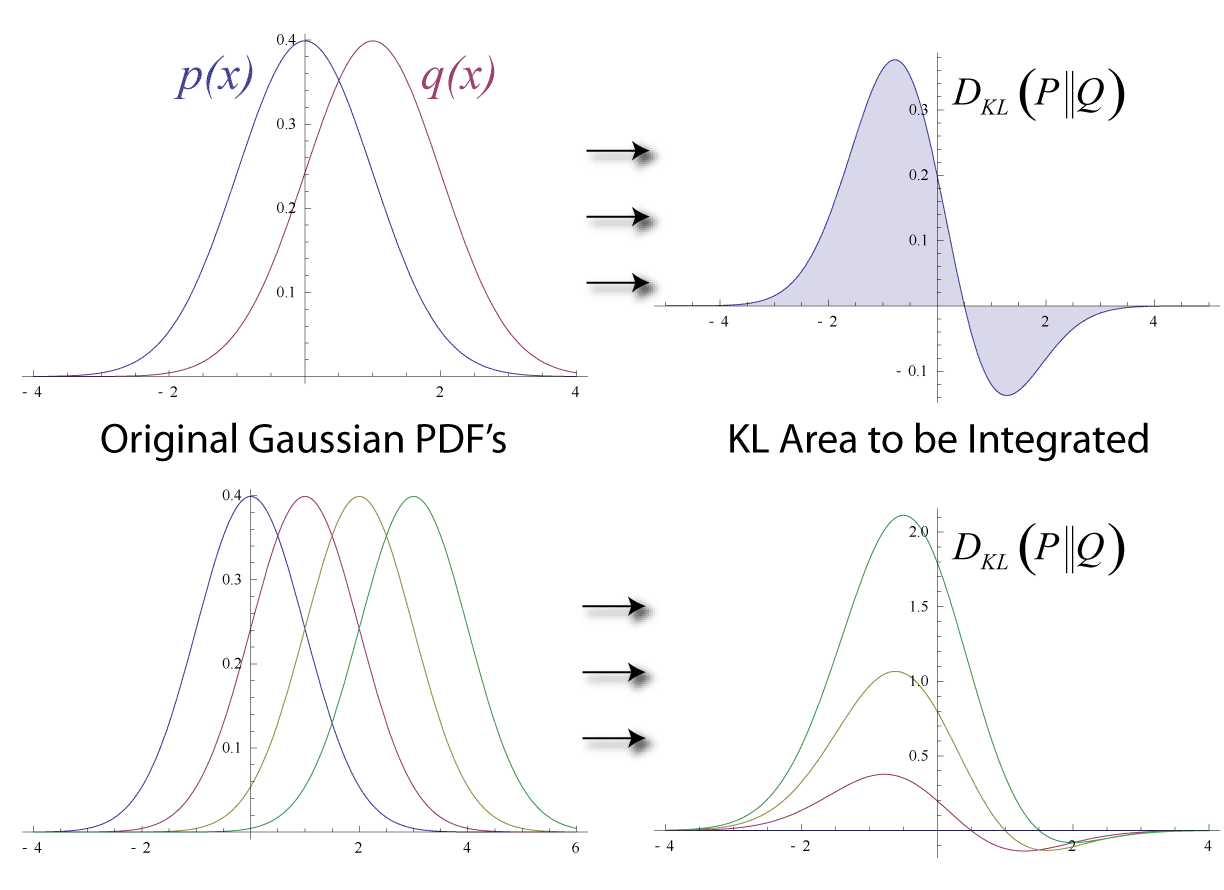$D_{KL} = D_{KL}\big({\it P}(A) || {\it Q}(B)\big)=\sum_{j=1}^{n} {\it P}(A=a_{j}) \log \Big(  \cfrac{{\it P}(A=a_{j})}{{\it Q}(B=b_{j})} \Big)$

$\log$ is in base $e$.

${\bf Definition}$: Mutual Information on Discrete Random Variates

Two discrete random variables $X$ and $Y$, having realisations $X=x_{k}$ and $Y=y_{l}$, over $m$ and $n$ singletons $k=1,...,n$ and $l=1,...,m$ respectively, are given. Mutual information, $I(X;Y)$ is defined as follows,

$I(X;Y) = \sum_{k=1}^{n} \sum_{l=1}^{m} {\it R}(X=x_{k}, Y=y_{l}) \log \Big(\cfrac{  {\it R}(X=x_{k}, Y=y_{l})  }{  {\it R}(X=x_{k})  {\it R}(Y=y_{l})} \Big)$

$\log$ is in base $e$ and $R$ denotes probabilities.

${\bf Definition}$: Mutual Information on Discrete Random Variates with KL distance

Two discrete random variables $X$ and $Y$. Mutual information, $I(X;Y)$ is defined as follows,

$I(X;Y) = D_{KL} \Big( {\it R}(X, Y) || {\it R}(X) {\it R}(Y) \Big)$

${\bf Problem: Example}$: Given two discrete random variables $X$ and $Y$ defined on the following sample spaces $(a,b,c)$ and $(d,e)$ respectively. Write down the expression for the mutual information, I(X;Y), expanding summation.

${\bf Solution: Example}$:

$I(X;Y)  = {\it R}(X=a, Y=d) \log \Big(\cfrac{ {\it R}(X=a, Y=d) }{  {\it R}(X=a)  {\it R}(Y=d)} \Big) +$

${\it R}(X=b, Y=d) \log \Big(\cfrac{  {\it R}(X=b, Y=d)  }{  {\it R}(X=b)  {\it R}(Y=d)} \Big)+$

${\it R}(X=c, Y=d) \log \Big(\cfrac{  {\it R}(X=c, Y=d)  }{  {\it R}(X=c)  {\it R}(Y=d)} \Big)+$

${\it R}(X=a, Y=e) \log \Big(\cfrac{  {\it R}(X=a, Y=e)  }{  {\it R}(X=a)  {\it R}(Y=e)} \Big)+$

${\it R}(X=b, Y=e) \log \Big(\cfrac{  {\it R}(X=b, Y=e)  }{  {\it R}(X=b)  {\it R}(Y=e)} \Big)+$

${\it R}(X=c, Y=e) \log \Big(\cfrac{  {\it R}(X=c, Y=e)  }{  {\it R}(X=c)  {\it R}(Y=e)} \Big)$


${\bf Definition}$: Two discrete random variables $X$ and $Y$, having realisations $X=x_{k}$ and $Y=y_{l}$, over $m$ and $n$ singletons $k=1,...,n$ and $l=1,...,m$ respectively, are given. Two-way contingency table of realisations of $X$ and $Y$ along the same measured data points, $N$ observations, can be constructed by counting co-occurances, or cross-classifying factors. Normalizing the resulting frequency table will produce joint and marginal probabilities.

                Y=y_{1}              ...    Y=y_{l}              R(X)
               
    X=x_{1}    R(X=x_{1}, Y=y_{1})   ... R(X=x_{1}, Y=y_{l}) R(X=x_{1})
    X=x_{2}    R(X=x_{2}, Y=y_{1})   ... R(X=x_{2}, Y=y_{l}) R(X=x_{2})
    ...             ...              ...        ...              ...
    X=x_{k}    R(X=x_{k}, Y=y_{1})   ... R(X=x_{k}, Y=y_{1} R(X=x_{k}) 
   
    R(Y)        R(Y=y{1})           ...  R(Y=y_{l})


Simulated example with R

1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18

set.seed(3951824) # Table of counts (contingency) X <- sample(letters[1:3], 100, 1) Y <- sample(letters[4:5], 100, 1) t <- table(X,Y) tp <- t/100 # proportions tn <- tp/sum(tp) # normalized, joints p_x <- rowSums(tn) # marginals p_y <- colSums(tn) P <- tn Q <- p_x %o% p_y # P(X, Y) : bin frequency: P_i # P(X) P(Y) : bin frequency, Q_i mi <- sum(P*log(P/Q)) library(entropy) mi.empirical(t) == mi

References

(KullbackLeibler57) Kullback, S.; Leibler, R.A. (1951). "On information and sufficiency". Annals of Mathematical Statistics 22 (1): 79–86.